Power Amplifier Circuit Diagram Problem

[TheAntiDoctor]

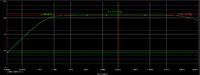
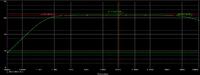
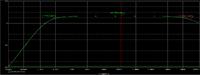
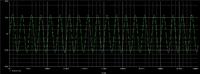
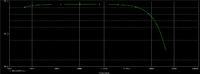
It is easy to measure the low frequency and high frequency -3dB points. EDIT: An audio amplifier is not measured up to 10GHz. I think this one is -3db at 80kHz that you cannot see on your very wide range of frequencies.

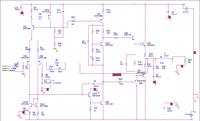
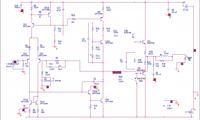
Maybe you should mention in your answer that this "blameless" amplifier was designed for 50W into 8 ohms and might be overloaded and might overheat with 110W into 4 ohms.

Thanks a lot for your speedy response, it's really appreciated! Thanks for the link to the article it was very useful and I had searched it before, however I hadn't realised that the component values were different from the ones he was referencing - hence I didn't immediately find the reference!

I'll be sure to mention the danger of overheating - it is a very crucial detail!

I had another go at measuring the bandwidth and came up with the attached images and corresponding traces. I calculated the bandwidth by finding the middle frequency and then the difference between the lower and upper bounds, despite changing the voltage from 0.1V to 1.414V the bandwidth changed very little, is this to be expected?

 

[Audioguru]

I had another go at measuring the bandwidth and came up with the attached images and corresponding traces.

Your graphs have numbers on the left side from 0 to 80. What are they? The left side of your graphs should be marked in decibels.
The gain of this amplifier is 21 times (26.444dB) so show the frequencies where the output has dropped -3dB.

The slew rate of the amplifier reduces its -3dB high frequency response when its output level is high. The instructions in your post #19 says to observe that the output is undistorted since the slew rate limit causes a sinewave to become a triangle wave.

 

[TheAntiDoctor]

Your graphs have numbers on the left side from 0 to 80. What are they? The left side of your graphs should be marked in decibels.
The gain of this amplifier is 21 times (26.444dB) so show the frequencies where the output has dropped -3dB.

The slew rate of the amplifier reduces its -3dB high frequency response when its output level is high. The instructions in your post #19 says to observe that the output is undistorted since the slew rate limit causes a sinewave to become a triangle wave.

I'm really sorry, I'm still a little bit confused. To recap:

1. So far I've set the input to 0.1V and measured the -3dB bandwidth using this method: http://www.sengpielaudio.com/calculator-cutoffFrequencies.htm. Then looking through the article provided by Audioguru I have determined the effect of the four components that make up the zobel network.

2. Now I need to take the upper bound from the bandwidth I just measured, about 50KHz and apply it as an input at 1.414V. Then inspect the output waveform for distortion?

 

[FvM]

To get the dBV quantity displayed as gain in the AC simulation, the voltage of an AC input source should be set to 1 VAC. But there's no AC source shown in the simulation circuit, so we can't know what the about 63 dBV number in the bode plot means.

 

[TheAntiDoctor]

To get the dBV quantity displayed as gain in the AC simulation, the voltage of an AC input source should be set to 1 VAC. But there's no AC source shown in the simulation circuit, so we can't know what the about 63 dBV number in the bode plot means.

Hi, thanks for taking the time to reply! I am not quite sure what you mean about there being no AC input to the simulation circuit. On the far left hand side there is an AC input of 0.1 V on the first example and 1.414V in the second example...

 

[FvM]

There's a SINE source, not an AC source. An additional AC value can be specified with the SINE source, so that it's acting as AC source in .AC analysis, but the value is apparently hidden in the symbol.

Obviously the VAC value used during AC simulation is not 0.1 or 1.44 V.

 

[TheAntiDoctor]

There's a SINE source, not an AC source. An additional AC value can be specified with the SINE source, but it's apparently hidden in the symbol.

Obviously the VAC value used during AC simulation is not 0.1 or 1.44 V.

Ah, now I understand! Thanks for taking the time to explain, I'll rerun the simulation with 1VAC. This probably seems like a stupid question, but what exactly is the difference between the SINE and AC source? I mean an AC source is just a sine wave, with the amplitude of the wave being the peak voltage. I'm sorry for the basic question, but I am completely new to Cadence and it can be hard to differentiate the many input sources available!

 

[FvM]

SINE source is used to get a sinoidal input voltage in transient simulation (time domain), AC source is used in .AC (small signal) simulation.

 

[TheAntiDoctor]

Hey guys,

Thanks a lot for your help so far, here are some updates and I'd love to hear if I'm going right. So I've rerun the simulation with an AC source at 0.1V attached are the schematics and the output trace. Then for the upper bound of the 0.1V input a 1V RMS input is applied at that frequency is an output waveform. If you could look at this and see if I've answered the question properly I'd really appreciate it.

I've also included the original question



- - - Updated - - -

The next question I'm coming up against this time is to do with measuring the flatness of the response. I believe I've done what they asked of me so I'd really appreciate it if you could just confirm that I've done it correctly.

I've input 1.414V (1V RMS) and then noted the change in voltage gain over the frequency range in dB for a range of about 0.8 dB

 

[Audioguru]

It is difficult or impossible to see -3dB on your frequency response curves unless you make the maximum at 0dB then use 1dB increments.

Your timebase (at the bottom) is too narrow so the sinewaves are crammed together which makes it difficult or impossible to see distortion. It calculates to be only 20kHz, not the upper -3dB frequency which is much higher.

 

[TheAntiDoctor]

Your timebase (at the bottom) is too narrow so the sinewaves are crammed together which makes it difficult or impossible to see distortion.

- - - Updated - - -

It calculates to be only 20kHz, not the upper -3dB frequency which is much higher.

Strange I know, but that's what was specified....

 

[FvM]

It is difficult or impossible to see -3dB on your frequency response curves unless you make the maximum at 0dB then use 1dB increments.

Fortunately, the OP did already mark the -3 dB points. Even without a cursor, you can read the corner frequencies with sufficient accuracy for a brief check.

I believe that the transient analysis waveform shows the response to the 40 kHz sine stimulation shown in the simulation circuit. There are no obvious crossover distortions, but to determine the amplifier performance in simulation exactly, you'll perform a fourier analysis at different sine levels and frequencies.

 

[TheAntiDoctor]

Fortunately, the OP did already mark the -3 dB points. Even without a cursor, you can read the corner frequencies with sufficient accuracy for a brief check.

Thanks a lot for your response! Audioguru's comments made me think that he was looking for the frequency points at the actual -3dB level rather than -3dB below the top frequency...

 

[FvM]

Yes, you should look for 3 dB gain drop, as correctly marked in post #29.

 

[Audioguru]

Your graphs do not say what they are. The numbers do not say is they if they are gain numbers or dBs.
One graph should say it is the output of the amplifier at a low level and the other graph should say at a high level.

I show one of your graphs with some questions that should be answered on the graph.
I show a typical graph of an amplifier's bandwidth showing 0dB at the maximum level and the frequencies where the response is at -3db.

You did not show a graph of where the bandwidth was limited by the slew rate and a fairly low distortion sinewave at that -3dB frequency. Instead you showed a horribly distorted 200kHz crammed waveform.
You show a graph where the maximum level is not even shown.

 

[TheAntiDoctor]

Your graphs do not say what they are. The numbers do not say is they if they are gain numbers or dBs.

You did not show a graph of where the bandwidth was limited by the slew rate and a fairly low distortion sinewave at that -3dB frequency. Instead you showed a horribly distorted 200kHz crammed waveform.
You show a graph where the maximum level is not even shown.

Hey, thanks for your reply and sorry for the confusion! :/

Unfortunately I am having to upload these as images and cadence doesn't really have too many options for adding things like that, but the graphs are in dB. This is indicated by the small notation in the corner of DB(V(R29:2)). With so much confusion it can be difficult to keep track of what's going on! I've removed the 1V measurements from post #29 this leaves the upper and lower -3dB levels from the 0.1V AC input.

The upper bound frequency I then plugged in at 1V RMS to the system to display the output sinewave, which according to FvM is undistorted.

I was just checking whether I had answered the tasks that I had been set correctly

- - - Updated - - -

I show one of your graphs with some questions that should be answered on the graph.
I show a typical graph of an amplifier's bandwidth showing 0dB at the maximum level and the frequencies where the response is at -3db.

Thanks a lot for taking the time to put that together for me, it's really appreciated!

I'm not sure whether the questions on the first graph are rhetorical or not, but yes those are dBs and those are 20Hz and 20kHz - the numbers that I was told to measure between to determine the flatness. As for the second thumbnail, that makes a lot more sense to me now so thanks for that, however I'm not sure how I could move the scale up and down without changing the input which I couldn't do and as I'm new to cadence I don't know how to move the trace down to make the maximum gain align with the 0dB marker. If the scale is linear, does it make a difference for readings?

Thanks for your time

 

[Audioguru]

I am sorry I was wrong. Since your post #29 sinewave frequency was not labelled, I wrongly calculated it as 20kHz instead of 40kHz. Sine the waveforms are crammed together it is difficult to see distortion.

Your bandwidth graphs in post #29 have 5dB increments instead of 1dB increments. It is difficult to see -3dB when the reference (the maximum level) is in between two 5dB lines.

 

[TheAntiDoctor]

Hi Guys,

So I'm finally coming to the end of my assignment, I have to hand in tomorrow morning, and there are just a few questions left that I'm having some difficulty with and I'd really appreciate it if you guys could give me some pointers with them, thanks again already for all the help that you've given!

OK so the last two questions involve measuring efficiency and harmonics. I know efficiency is to do with the ratio of input to input in principle but I have no real idea of how to apply this principle to an audio amplifier and I am running dangerously low on time. The second question is to do with measuring distortion and harmonics. I have measured the voltage output of the circuit at the specified input (attached along with the original questions) but I'm not sure how to get the harmonics.

Another task that I've been set is to estimate the cost of the amplifier, the power supply components and packaging costs of the amplifier. I'm really not sure where to even begin with that one!

Again, any suggestions however small are gratefully recieved, thanks for taking the time to read the post!

 

[Audioguru]

Your sinewaves are crammed together again because the timebase at the bottom is 10ms across the screen instead of 2ms.

Efficiency is Power Out/Power In.
You have an output that is 60V p-p into 4 ohms so the RMS voltage is 60V/2.828= 21.2V RMS. Your load is 4 ohms so the output power is (21.2V squared)/4 ohms= 112.4W.
Power In is the supply voltage (74V p-p) times the supply current that you measure at the amplifier or in your simulation.
I guess your amplifier is about 60% efficient at 112.4W output.

I use a distortion analyser to measure distortion. In a simulation program you can measure distortion directly and you can do an FFT test to show the harmonics and their levels.

Look up the cost of the components and guess at the packaging costs.