CataM
Advanced Member level 4

- Joined
- Dec 23, 2015
- Messages
- 1,275
- Helped
- 314
- Reputation
- 628
- Reaction score
- 312
- Trophy points
- 83
- Location
- Madrid, Spain
- Activity points
- 8,409
Yes, I figured out FvM was referring to a single wire afterwards.
I was initially posting about "transformer windings" where it is assumed windings are wound in layers and hence only 1D H field is possible, hence Dowell expression is somehow accurate.
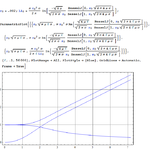
The strange thing is Easy Peasy's claim of 15% rise of their Rac with respect to Rdc with the claimed wire size. Obviously he is hiding some details.
I was initially posting about "transformer windings" where it is assumed windings are wound in layers and hence only 1D H field is possible, hence Dowell expression is somehow accurate.
The strange thing is Easy Peasy's claim of 15% rise of their Rac with respect to Rdc with the claimed wire size. Obviously he is hiding some details.