yasemin_
Newbie level 5

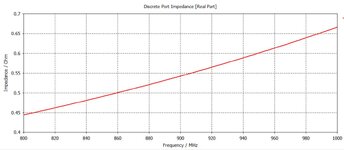
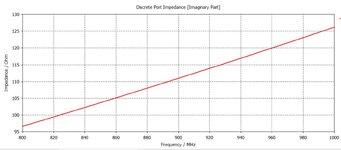
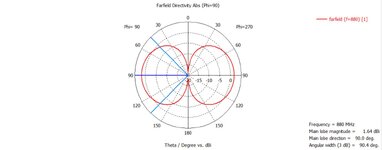
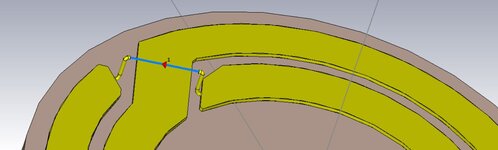
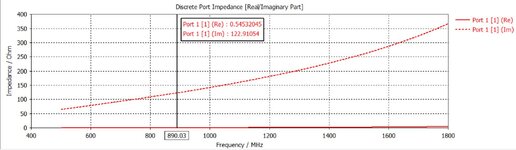
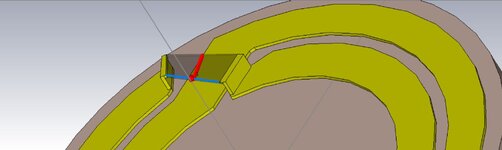
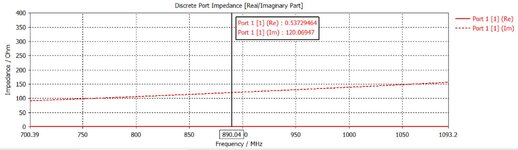
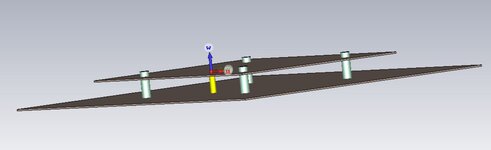
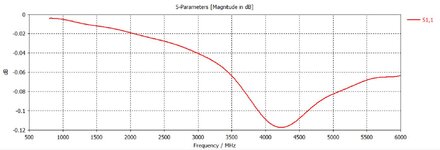
Hello, I am trying to simulate an RFID tag in CST. The structure in the image I attached is to match the impedance of the chip. I am trying to find the input impedance of this with simulation. I simulated it using discreate port but the impedance value I got is incorrect. Am I using the port wrong? The real component of the impedance I need to get for around 890 MHz is 0.22 ohms, the imaginary component is 120 ohms. But what I get is different. In addition to that I should get an omnidirectional radiation pattern, but this is also different. I can't find where I made a mistake.