imar
Full Member level 1

difference between positive and negative feedback
hi freinds;
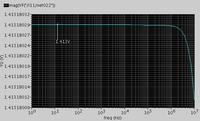
we have spoken later in (Problem with LDO design) about the positive and the negative feedback for the error amplifier and hopefully as LvW said, the PM is not always calculated by taking the origin in -180° and LVW has well explained it.
so at this point, a question could be put: what is the difference between having a positive feedback or a negative one? how could we choose between them?
in what point does this feedback affect ?
thanks for information in advance!
regards
hi freinds;
we have spoken later in (Problem with LDO design) about the positive and the negative feedback for the error amplifier and hopefully as LvW said, the PM is not always calculated by taking the origin in -180° and LVW has well explained it.
so at this point, a question could be put: what is the difference between having a positive feedback or a negative one? how could we choose between them?
in what point does this feedback affect ?
thanks for information in advance!
regards