hioyo
Advanced Member level 4

I was learning about phasor analysis from Fundamentals of electric circuits by Alexander and Sadiku.
In chapter 9 the below trigonometric identity is given.

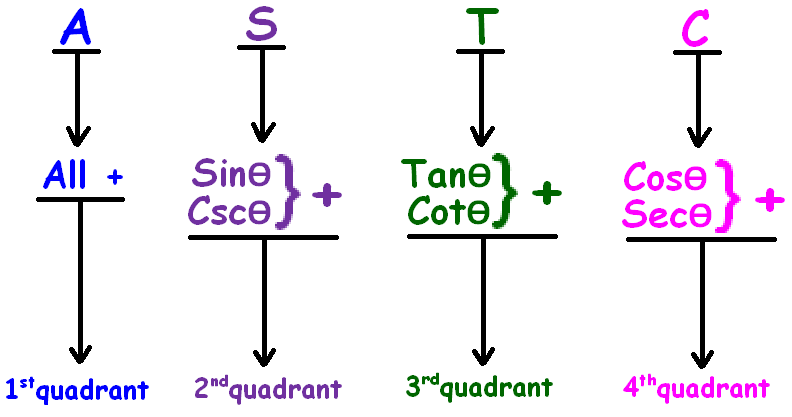
As per my knowledge sin is positive in the 2nd quadrant and negative in all other quadrants(ALL SILVER TEA CUPS). In the first equation Sin(wt±180) means it is lying in the 2nd quadrant and 3rd quadrant depending phase shift is +180 or -180.
When it is lying in the 2nd quadrant**(Sin(wt-180))**it should be positive and when it is lying in the 3rd quadrant **(Sin(wt+180))**should be negative.This is my understanding.
But in the book it is given as Sin(wt±180)=-sinwt .May I know where I went wrong
In chapter 9 the below trigonometric identity is given.
As per my knowledge sin is positive in the 2nd quadrant and negative in all other quadrants(ALL SILVER TEA CUPS). In the first equation Sin(wt±180) means it is lying in the 2nd quadrant and 3rd quadrant depending phase shift is +180 or -180.
When it is lying in the 2nd quadrant**(Sin(wt-180))**it should be positive and when it is lying in the 3rd quadrant **(Sin(wt+180))**should be negative.This is my understanding.
But in the book it is given as Sin(wt±180)=-sinwt .May I know where I went wrong