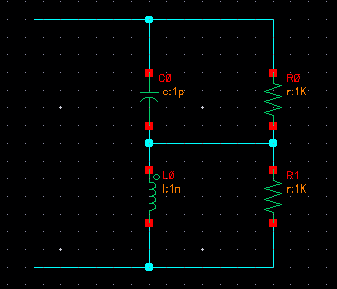
LvW, thank you so much for your detailed reply. I suppose I was getting caught up in the definition of characteristic impedance as it pertains to transmission lines, but the math that you have described makes perfect sense. I am actually pursuing this problem as a way to calculate the input impedance of the circuit attached here:

Using what you've described, I calculate Rin as 2R/[1+R/Z0], although...I have also seen it as 2R/[1+(R/Z0)^2]?? I must be making a mistake somewhere in the calculation.