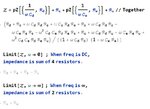kareno
Newbie level 4

Follow along with the video below to see how to install our site as a web app on your home screen.
Note: This feature may not be available in some browsers.





"w" still includes frequency, since w = 2 x Pi x fIf I use w instead of frequency, I am wondering this is correct.
I still don't understand. How is voltage involved in impedance calculation?Eliminating the voltage meant that I could ignore the voltage when calculating the impedance.


It is very easy thing.I am wondering how to handle the voltage.

It is very easy thing.
Your problem is no more than Thevenin Equivalent circuit theorem.

I am not sure because it is confusing.


Like the above calculation?



The usual tactic is to imagine all supply rails are 0v. Then calculate time constants, series branches, parallel branches, etc. Because time constants don't change regardless of supply voltage, whether capacitors are charging or discharging.
The top and bottom ends of your schematic should have a wire joining them, if that's the entire circuit.

The picture is how I calculated it

Does that mean that the impedance cannot be obtained because this circuit does not represent the whole part? If I give the value of each resistor or capacitor, can I find the impedance?

The total impedance was calculated using the capacitor's impedance of 1 / jwc and the resistance of R.
The picture is how I calculated it.View attachment 158778

Does that mean that the impedance cannot be obtained because this circuit does not represent the whole part? If I give the value of each resistor or capacitor, can I find the impedance?

I want to find the impedance in this circuit.
Is it possible to calculate the capacitor as 1 / jwc after removing the voltage from this circuit?
I am not sure because it is confusing.
Thank you for letting us know how to do the calculations with and without the dashed part of the circuit.View attachment 158756