Can momentum give me the power gain from one anntenna to another?

[grit_fire]

Hi, all,

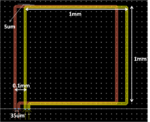
My goal is to find the power gain transferring from one antenna (transmitter) to another (receiver). I drew two antennas. One strange thing occurs to me is that momentum never asks me to clarify the transmitter and receiver. After simulation, I obtained s11, s12, s21, s22.

Can I know my power gain based on these parameters?

Is momentum treating one of them as transmitter and another as receiver automatically? Or my bottomline question is: can momentum give me power gain of the 2-antenna system at all??

Thank you for answering my question!

Best,
grit_fire

 

[WimRFP]

Hello,

The S parameters fully characterize your antenna configuration as a black box. I assume you have same source impedance on both sides. If you use different antennas, S11 may differ from S22, but S21 = S12.

S-parameters are defined based on voltages, so:

(received power at port 2)/(incident power at port 1) = |S21|^2 = (received power at port 1)/(incident power at port 2) = |S12|^2

As you have a passive system, the result should be < 1, but greater then zero (so in dB's the power gain must show a negative value).

If you have large mismatch between source or load and the antennas (this means large S22 and/ or S22), you may add some matching. This will increase the power transfer between the antennas.

If you are familiar with VSWR: VSWR = (1+|S11|)/(1-|S11|). Mismatch loss = -10*log(1-|S11|^2)
you may also use S22 for the receive antenna.

Note that the mismatch formula only gives useful results in case of a linear behaving source. When using a real power amplifier, the loss may increase.

If you use same antennes for both sides, and know the distance, you can calculate the antenna gain with the Friis formula (as Gtx = Grx in that case). Don't forget to check for far field (Fraunhofer region) conditions.

 

[grit_fire]

thanks a lot for your reply!

I am using the two identical antennas. I don't have source or load for them. I am simulating in layout editor of momentum. My schematic only contains two antenna and each of them have two differential terminals. That's all.

Can I use |S21|/|S12| which is the maximum stable gain in this case to calculate the power gain? Is it eligible to use here?

Thank you

 

[WimRFP]

Hello,

As there is no active component in your circuit, there is no change on instability (parasitic oscillation), for every combination of source and load, the power gain of your two-antenna setup will be less then 0 dB. Therefore, you will not encounter |S11| or |S22| greater then one.

The source and load impedance are defined in the S-parameter setup (I assume default 50 Ohms). For a differential feed, the default values can be different. Perhaps other people can give useful info on this (if this is of interest).

 

[grit_fire]

Hi, WimRFP,

Thanks for the reply!

I am not sure if I understand what you meant. Were you suggesting that |S12|/|S21| won't be applicable here? Because from my simulation, S21=S12 and hence |S12|/|S21|=1 which means 100% efficiency. This won't make sense, right?

So I should just use |S12|^2? Is that what you meant?

Thank you!!

Hello,

As there is no active component in your circuit, there is no change on instability (parasitic oscillation), for every combination of source and load, the power gain of your two-antenna setup will be less then 0 dB. Therefore, you will not encounter |S11| or |S22| greater then one.

The source and load impedance are defined in the S-parameter setup (I assume default 50 Ohms). For a differential feed, the default values can be different. Perhaps other people can give useful info on this (if this is of interest).

 

[WimRFP]

Hello,

The ratio of |S21| and |S12| has to to do with the stability of electronic components/circuits, just use |S21|^2 to find the power transfer between the antennas.

The maximum power transfer (after matching both load and source) would be: |S21|^2/{ (1-|S11|^2)*(1-|S22|^2) }. Assuming |S21| << 1.

From the above formula, you can see that when |S11| and |S22| are well below 1 (that means low relfection), you can use |S21|^2 (or |S12|^2 ) as a good approximation for the maximum power transfer.

 

[grit_fire]

Hi, Thanks!

But I'd doubt if there might be an error in the formula. If we do a unit checking, the nominator gives Watt but won't the denominator gives watt^2?

Hence, I suspect that you meant |S21|^2/(1-|S11|^2) only?

Thank you!

Hello,

The ratio of |S21| and |S12| has to to do with the stability of electronic components/circuits, just use |S21|^2 to find the power transfer between the antennas.

The maximum power transfer (after matching both load and source) would be: |S21|^2/{ (1-|S11|^2)*(1-|S22|^2) }. Assuming |S21| << 1.

From the above formula, you can see that when |S11| and |S22| are well below 1 (that means low relfection), you can use |S21|^2 (or |S12|^2 ) as a good approximation for the maximum power transfer.

 

[WimRFP]

Hello,

You are correct with respect to the dimensions. The complete formula is longer, but when |S21|*|S12| << 1 and |S11|, |S22| << 1, the input reflection of port 1 virtually doesn't change with changing load at port 2 (and vice versa). For losless networks, you need to use the complete formula.

If both |S11| and |S22| are small (say below 0.33, VSWR=2) and |S21| is even smaller (mostly the case with radio antennas), you can ignore the corrections (worst case error < 1dB) .

 

[grit_fire]

Thank you so much for the help! WimRFP!

Now there is a new problem. As I completed several simulations with different thickness of the substrate. Some weird result came out.

Sometimes the S11 and s22 are several dB above zero. This doesn't make sense, right? I would have a negative power gain if using |s21|^2/(1-|s22|^2). I am not sure if one of my settings is incorrect or I missed to set something. What could possibly cause this??

Also, as what mesh frequency should I choose, It seems each time I will obtain different S parameter values for different mesh frequencies I input.

I appreciate your help!

 

[WimRFP]

Hello,

input/output reflection is frequently expressed in dB. It can have a form of 20*log(|S11|). This should give a negative or zero number for passive circuits.

so |S11| = 0.333, should give -9.5 dB. With this definition, you shouldn't get positive dB numbers. You may change your display to smith chart, and check S11 on the graph, it should always be in the passive region (so |S11| < 1)

You may also encounter the Return Loss (the loss of the reflected signal). This is defined as RL = -20log(|S11|) (note the minus sign). For a passive network, RL >= 0. In cable and antenna related stuff you will see this definition frequently.

Note that for many two ports, the actual input reflection (so (reflected voltage)/(reverse voltage) may not equal S11, as actual input reflection coefficient may depend on the reflection coefficient of the load.

So generally spoken: RL = -20*log(|input reflection coefficient|) .

|S21|^2/(1-|S22|^2) should give a positive number less then one for your antenna case.

When expressed in dB's: 10*log{|s21|^2/(1-|s22|^2)} should give a negative number. Note the "10" as the formula itself is a power ratio already.

Regarding the meshing frequency, this depends on how fast versus distance the current density may change. As a wild guess, you may start with a mesh size of about lambda/20, based on the wavelength in the substrate. At a certain point, reducing the mesh size will not result in changed simulation results (but very long run times).

If you have some interpolation scheme active, make sure you have sufficient frequency points. Interpolation schemes may give weird results.

Do you have a plot/graph of what you are simulating?

 

[grit_fire]

Hi, Thank you!

1) Another weird thing just happened. When I shift the antenna with ports labelled with smaller numbers (i.e. port1 and port2) to the left of the antenna with bigger numbers (i.e. port 3 and 4), (previously I had one exactly on top of the other) and then I simulated it again. The s11 turned out to be less than 1 again! I really had no idea why changing the relative position of two antennas could affect the s11 and s22 to be greater or less than 1! Do you have any idea why that happened?

2) Another thing is that: how do I know if I have simultaneous conjugate matching in my system? It seems that I can only define the impedance for the port to be 50Ohms by default. Is that the load impedance? how about source impedance and transmission line characteristic impedance? Where can i view them?

Thank you!!

Hello,

input/output reflection is frequently expressed in dB. It can have a form of 20*log(|S11|). This should give a negative or zero number for passive circuits.

so |S11| = 0.333, should give -9.5 dB. With this definition, you shouldn't get positive dB numbers. You may change your display to smith chart, and check S11 on the graph, it should always be in the passive region (so |S11| < 1)

You may also encounter the Return Loss (the loss of the reflected signal). This is defined as RL = -20log(|S11|) (note the minus sign). For a passive network, RL >= 0. In cable and antenna related stuff you will see this definition frequently.

Note that for many two ports, the actual input reflection (so (reflected voltage)/(reverse voltage) may not equal S11, as actual input reflection coefficient may depend on the reflection coefficient of the load.

So generally spoken: RL = -20*log(|input reflection coefficient|) .

|S21|^2/(1-|S22|^2) should give a positive number less then one for your antenna case.

When expressed in dB's: 10*log{|s21|^2/(1-|s22|^2)} should give a negative number. Note the "10" as the formula itself is a power ratio already.

Regarding the meshing frequency, this depends on how fast versus distance the current density may change. As a wild guess, you may start with a mesh size of about lambda/20, based on the wavelength in the substrate. At a certain point, reducing the mesh size will not result in changed simulation results (but very long run times).

If you have some interpolation scheme active, make sure you have sufficient frequency points. Interpolation schemes may give weird results.

Do you have a plot/graph of what you are simulating?

 

[bleuame]

do you understand the gain of antenna? and the power gain?

The gain of antenna is different to amplifer or passive element.

The gain of antenna is relative value of omni antenna.

FYI.

 

[WimRFP]

Hello,

Point 1. I am not fully clear of what you mean, do you have a drawing or screen plot?

Point 2. For a real source impedance, you have conjugate match when S11 is (almost) zero (with your impedance as reference for the S-parameters). How close it must be to zero depends on your application. Most antennas for telecom applications have VSWR<2 that equals |S11| < 0.333.

For non-real sources, I think you can't get it directly from the simulation as the S-parameters are defined for real impedances only. If your complex source impedance is a + bj, the required load impedance for conjugated match (equals maximum power transfer) = a - bj .

 

[grit_fire]

Hi,

1) Attached is the layout that works. So the yellow one (with port 3&4) has to be to the right and below the red one (with port 1&2) in order to get a S11 less than 0.

2) How do i view my complex source impedance?

Thank you!

Hello,

Point 1. I am not fully clear of what you mean, do you have a drawing or screen plot?

Point 2. For a real source impedance, you have conjugate match when S11 is (almost) zero (with your impedance as reference for the S-parameters). How close it must be to zero depends on your application. Most antennas for telecom applications have VSWR<2 that equals |S11| < 0.333.

For non-real sources, I think you can't get it directly from the simulation as the S-parameters are defined for real impedances only. If your complex source impedance is a + bj, the required load impedance for conjugated match (equals maximum power transfer) = a - bj .

 

[tamoor]

juz a question came in mind , if i use 4 omni antennas in one building, will they cause interference due to radiation? all 4 antanna working on orthogonal channels.

 

[WimRFP]

Hello,

What you are doing is completely off what I had in my mind; you are in near field (reactive field) communication. What is the distance between the layers and frequency?

I assume there is ground present (because of the four ports). You may consult the manual/help file for details of the feed schemes as this has large influence on usefulness of the results. .

Ports with respect to ground that use de-embedding, you can't put on top of each other (maybe some momentum expert can confirm this?).

Other thing is when you have conductors very close to each other, current may concentrate at certain regions. The meshing has to be fine enough to enable simulation of steep current density changes versus distance.

Regarding source impedance, I don't know the circuit that will actually drive these antennas. For good functioning, your electronic circuits need to see certain impedance. For the TX case this is to assure certain power transfer, circuit stability, efficiency, etc. For RX it is mostly a Noise Figure or stability issue.

---------- Post added at 23:16 ---------- Previous post was at 23:14 ----------

juz a question came in mind , if i use 4 omni antennas in one building, will they cause interference due to radiation? all 4 antanna working on orthogonal channels.

With interference, do you mean that the 4 channels will interfere each other (mutually), or that the combined radiated field of the 4 channels will interfere in some other system (EMC issue)?

Can you further describe "orthogonal" (is this in frequency, time or spreading sequence, etc)?

When you limit yourself to metallic antennas, antennas and propagation are linear, obey superposition, but can be strongly time variant.

 

[grit_fire]

Hi,

The distance between the two layers is 10cm and frequency I simulated is 403 MHz to 405 MHz. Hence, indeed, the operation is in near field.

There is no ground. Two antennas are placed in free space and their ports are all differential ports.

Will the simulation results makes sense in this case?

Hello,

What you are doing is completely off what I had in my mind; you are in near field (reactive field) communication. What is the distance between the layers and frequency?

I assume there is ground present (because of the four ports). You may consult the manual/help file for details of the feed schemes as this has large influence on usefulness of the results. .

Ports with respect to ground that use de-embedding, you can't put on top of each other (maybe some momentum expert can confirm this?).
\oint
Other thing is when you have conductors very close to each other, current may concentrate at certain regions. The meshing has to be fine enough to enable simulation of steep current density changes versus distance.

Regarding source impedance, I don't know the circuit that will actually drive these antennas. For good functioning, your electronic circuits need to see certain impedance. For the TX case this is to assure certain power transfer, circuit stability, efficiency, etc. For RX it is mostly a Noise Figure or stability issue.

---------- Post added at 23:16 ---------- Previous post was at 23:14 ----------



With interference, do you mean that the 4 channels will interfere each other (mutually), or that the combined radiated field of the 4 channels will interfere in some other system (EMC issue)?

Can you further describe "orthogonal" (is this in frequency, time or spreading sequence, etc)?

When you limit yourself to metallic antennas, antennas and propagation are linear, obey superposition, but can be strongly time variant.

 

[tamoor]

With interference, do you mean that the 4 channels will interfere each other (mutually), or that the combined radiated field of the 4 channels will interfere in some other system (EMC issue)?

Can you further describe "orthogonal" (is this in frequency, time or spreading sequence, etc)?

When you limit yourself to metallic antennas, antennas and propagation are linear, obey superposition, but can be strongly time variant.



well i mean both, their mutual interference and their combined interference to main antenna, and orthogonal in terms of frequency.

Thank you

 

[tamoor]

power gain would be different of both antennas, i can set anything.

 

[WimRFP]

Hello Grit_fire,

I don't know momentum, but if you use the differential feed within the limitations, the simulation results should be OK (I expected that a differential feed based on 2 separate ports requires a ground plane). Did you also try two localized ports?

Other question may be: Does this simulation represent your real world antenna problem? How are you going to connect these small loops to your system? Think of: via coaxial cable, via coaxial cable with balun, via balanced lines (PCB traces) and balun function at input/output of TX/RX circuit, etc. The actual feed method may introduce common mode current on the feed line. If this happens this will affect the S-parameters, even when referenced at the input of the two loops.

What is the actual application for these small antennas?


Hello Tamoor,

I still think I don't understand what you mean, can you further clarify your question/problem?