adding ressistor on the output to cure problematic slope in bode plot

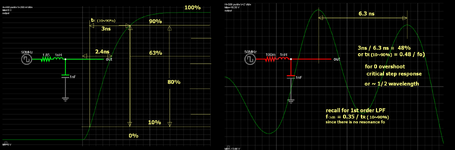
[yefj]

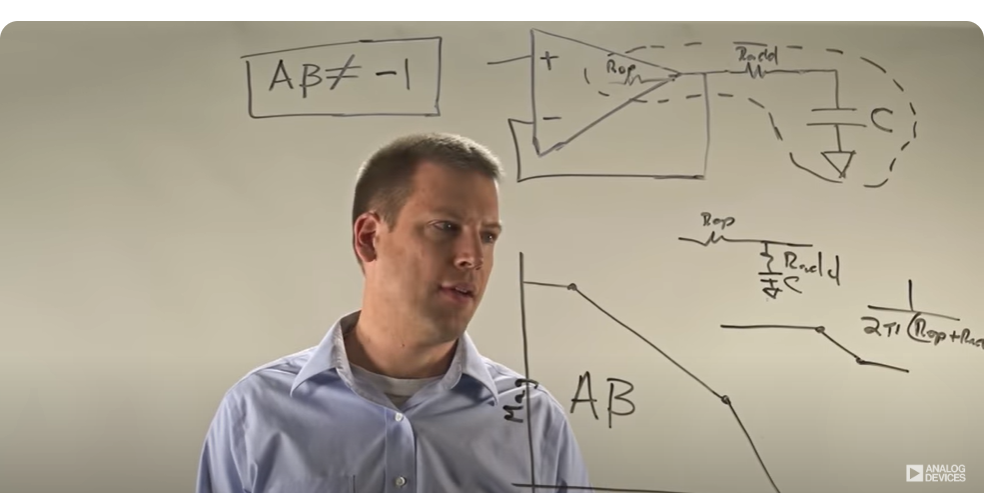
Hello , on the third minute of the manual shown in the link at minute 3 he says that when we add a resistor our added pole is 1/(Rop+Radd)C
Then he says that when the impedance of the capacitor in the same order ar the added resistor then we have a ZERO effect and the slope gets less steep.
How does a pole can turn to zero?
What is the math behind it?
Thanks.

 

[D.A.(Tony)Stewart]

http://web.mit.edu/2.14/www/Handouts/PoleZero.pdf

When Impedance of C matches series Rs then you are near critical damping.
My RLC impedance 4D graph helps look up these values.

e.g. here L=C=1e-9 and Zo=sqrt(L/C)= 1 so adding Rs=1 make it "near" critically damped with a very low peak in frequency response and shifted slightly lower. (Q = 1⁄2) is said to be critically damped.

There are tables with direct correlation to 2nd order Q and overshoot.

Wikiwand - Q factor

In physics and engineering, the quality factor or Q factor is a dimensionless parameter that describes how underdamped an oscillator or resonator is. It is defined as the ratio of the initial energy stored in the resonator to the energy lost in one radian of the cycle of oscillation. Q factor is...

www.wikiwand.com

 

[danadakk]

If we ignore the internal z's of the OpAmp output, we have a series
R and C to ground, so

Z = R + 1/sC = (RCs+ 1) / sC = [ RC (s + 1/RC) ]/sC, hence you can see the zero
in numerator.


Regards, Dana.

 

[D.A.(Tony)Stewart]

If we ignore the internal z's of the OpAmp output, we have a series
R and C to ground, so

Z = R + 1/sC = (RCs+ 1) / sC = [ RC (s + 1/RC) ]/sC, hence you can see the zero
in numerator.


Regards, Dana.

but at unity gain frequency then internal Z of op Amp may be relevant if R approaches Zo.

e.g. Op Amp Zo may vary from 20 to 250 ohms in BJT type

So for critical damping step response, include internal Zo and choose Rtotal = 1.85 to 2 * X(f) at breakpoint, or fo resonance

It depends if you want slightly faster step response with overshoot, or no overshoot.
But recall Vin+ always has greater BW.