lhlbluesky
Banned

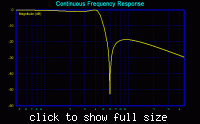
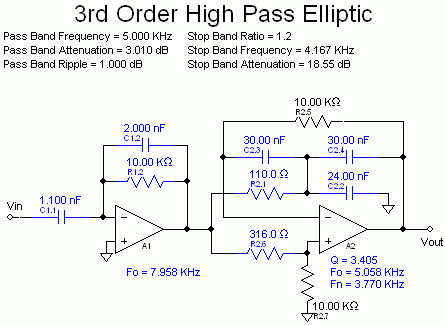
i'm a junior for active filter design. i will design a band-pass filter, the input signal is noisy, and my filter must have very good performance of attenuating the noise in both side. the center frequency f0 is 20Khz, Q must be larger than 8, and f0 must be accurate enough(tolerance 2%) for process and temp variation. i was told that, the design is difficult, i have checked some papers, but have no ideas yet, so, pls help me, and tell me what circuit structure should i used? i want it to be 2-order and using one opamp, can this be ok?
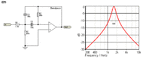
besides, i want to ask another question, for active filter (such as twin-t filter), how to decide and calculate the parameter of f0, Q, BW, gain? how to get the formula of them for a complicated transfer function (2-order or high order)? for 2-order filter, i know there is a typical formula just as :
vo/vi=A*w0/Q*s/(s^2+w0/Q*s+w0^2), but for a complicated transfer function or high order (4-order or 5-order) active filter, how to get the formula of f0 and Q? and what is the relationship of these parameters with opamp dc-gain?
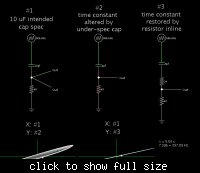
if i want to make f0 accurate enough(tolerance 2%) , what methods should i use? and how to do trim for f0 control?
thanks all. pls help me.
besides, i want to ask another question, for active filter (such as twin-t filter), how to decide and calculate the parameter of f0, Q, BW, gain? how to get the formula of them for a complicated transfer function (2-order or high order)? for 2-order filter, i know there is a typical formula just as :
vo/vi=A*w0/Q*s/(s^2+w0/Q*s+w0^2), but for a complicated transfer function or high order (4-order or 5-order) active filter, how to get the formula of f0 and Q? and what is the relationship of these parameters with opamp dc-gain?
if i want to make f0 accurate enough(tolerance 2%) , what methods should i use? and how to do trim for f0 control?
thanks all. pls help me.