[circuit theory] Transfer function of feedback network

[melkord]

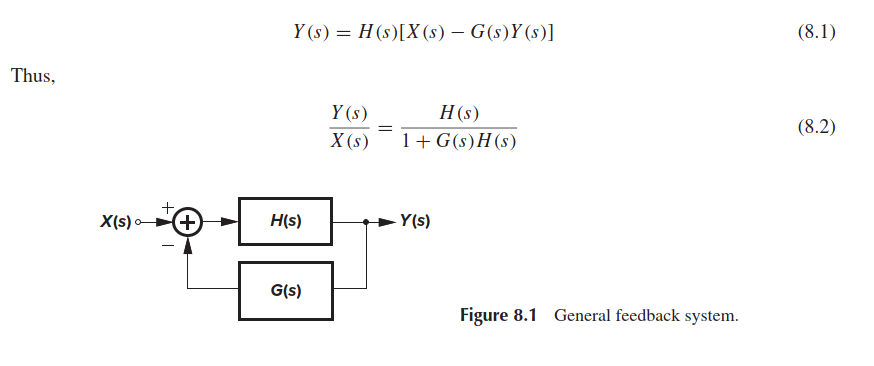
In the system with negative feedback, what is described by G(s) actually? is it G(s)=A(s)/Y(s) ?

If I want to derive Y(s)/X(s), is it a correct way to derive G(s)=A(s)/Y(s) and then I can plug it in to the equation (8.2)?

With simple resistor as feedback, I usually derive the transfer function directly.
But right now, I have a quite complicated feedback network with some frequency-dependent components.
That's why I want to derive G(s) first.

 

[danadakk]

Just derive the G(s) element expression, and plug that
into 8.2, just as you do for H(s).

The knowns are H(s), G(s), X(s)......ie you know what sig in is, you
know what H(s) and G(s) are,.....

Signal flow graphs are great for doing this, see attached.

http://www.ittc.ku.edu/~jstiles/723/handouts/section_4_5_Signal_Flow_Graphs_package.pdf

Signal Flow Graph - Chrome Web Store

Signal Flow Graph Solver Application

chrome.google.com

Regards, Dana.

 

[melkord]

Just derive the G(s) element expression, and plug that
into 8.2, just as you do for H(s).

sorry it is a bit unclear for me.
I think I should derive G(s)=A(s)/Y(s) when the feedback network is detached from the feedforward. Is this correct?

I will check your links. Thanks

 

[danadakk]

Yes, you treat G(s) as a stand alone network with an input
and output. But dont use Y(s) as input because that would make
it a f( G(s), X(s), Y(s) ). So make input Z(s), output A(s), and then
solve for is T(s) = A(s) / Z(s) and plug that into the general T(s)
for the overall problem.

Regards, Dana.

 

[FvM]

I wonder what you mean with "derive G(s)"? The feedback factor is intentionally chosen in the design process.

 

[melkord]

I wonder what you mean with "derive G(s)"?

deriving the transfer function from small signal model.

The feedback factor is intentionally chosen in the design process.

I am on the brink of design process....still deriving the transfer functions.

 

[FvM]

You are stating in post #1:

I have a quite complicated feedback network with some frequency-dependent components.
That's why I want to derive G(s) first.

In this case, G(s) will be described by a rational function with numerator and denominator coefficients (zeros and poles). Consider that the "detached" feedback network must be loaded similarly to the final circuit.

 

[FvM]

As additional remark, I'd suggest an extended feedback scheme with separate forward transfer function F(s). It gives more options to design physical plausible feedback amplifier models.

There is a number of previous threads discussing feedback circuit descriptions, e.g.: https://www.edaboard.com/threads/how-to-determine-feedback-factor.174717