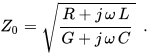Is EM at very low frequency like 20Hz propagates slower?

[Alan8947]

I read a paper attached here that claimed speed of the EM wave is slower at low frequency. It claimed at 20Hz, the speed is 500,000,000m/s vs the more conventional about 1,100,000,000m/s of higher frequency and RF. I never studied EM at this low frequency and I never see anything in textbooks that talk about this. Is there any truth in the paper?

Thanks

 

[FvM]

Although the article quotes several physical facts correctly, the conclusions make no sense to me. And they are completely different from my observations of actual cable behaviour at low frequencies. Propagation speed of electrical signals in coaxial cable is basically c/√Er, almost frequency independent in the discussed range.

- - - Updated - - -

A necessary supplement. The usual assumption of frequency independent impedance and propagation delay of coaxial or twisted pair cables is valid as long as the series impedance is dominated by characteristic inductance. Below a certain corner frequency, cable resistance becomes dominant. Characteristic impedance rises and propagation speed drops below this frequency. In so far the curves in the article are correct.

But the article misses to quantify the effect, e.g. for an audio cable system. It conveys an impression there can be audible effects without describing it explicitly.

The actual purpose behind this article is cable marketing, targeting to an esoteric high end audio scene.

 

[Alan8947]

Although the article quotes several physical facts correctly, the conclusions make no sense to me. And they are completely different from my observations of actual cable behaviour at low frequencies. Propagation speed of electrical signals in coaxial cable is basically c/√Er, almost frequency independent in the discussed range.

- - - Updated - - -

A necessary supplement. The usual assumption of frequency independent impedance and propagation delay of coaxial or twisted pair cables is valid as long as the series impedance is dominated by characteristic inductance. Below a certain corner frequency, cable resistance becomes dominant. Characteristic impedance rises and propagation speed drops below this frequency. In so far the curves in the article are correct.

But the article misses to quantify the effect, e.g. for an audio cable system. It conveys an impression there can be audible effects without describing it explicitly.

The actual purpose behind this article is cable marketing, targeting to an esoteric high end audio scene.

Thanks for your reply, can you give me a link(s) on the equation that show when cable resistance becomes dominant, the characteristic impedance rises and propagation speed drops at very low frequency? I never seen anything talk about this in books. But of cause, I mainly concentrate in RF and microwave side of EM, never even get in audio frequency range.

Thanks

 

[Nik_2213]

The 'High End Audio' scene is weird...

'Highest Fidelity' audiophiles will happily buy and fit hugely expensive 'triple gold plated' jumper cables -- For a digital link !!

I'm convinced that much is 'Placebo Effect', but YMMV.

Due Care, Please !!

 

[Alan8947]

Yes, I know better than that. I am just interested in EM theory and I never think about EM in audio frequency. I am more interested in learning.

I did a quick calculation, even if I accept the speed of EM in coax is 5EE6m/s, at 20Hz, I calculated the propagation is about 1.44deg going through a 1m long cable. That's not much of a phase shift. Usually interconnect cables are less than 1m long ( I won't use longer than that if I can help it.) as the capacitance of the cable do go up. It's about 30pF/ft, the longer the cable, the more the shunt capacitance. For old tube equipment, output impedance is higher, it might affect the high frequency response ( again, that's also a stretch).

Also, more importantly, even if it is true the velocity slow down to 5EE6m/s and phase delay is 1.44deg/m for 20Hz. This is compare to a PERFECT situation where the velocity of propagation is the same at all frequency.

BUT, this is a cable comparison, meaning it is compare one cable to another, not comparing a cable to perfect condition of equal velocity. The question remains how much one can improve the velocity on the best cable material compare to the average cable. Say, if the best of the best cable only improved to say 1deg/m, that improvement is only 0.44deg/m difference between the normal coax to the best of the best coax they are trying to sell. The difference is even smaller.

I just more interested in finding out the formula that show me the speed is slower at 20Hz.

 

[KlausST]

Hi,

I did a quick calculation, even if I accept the speed of EM in coax is 5EE6m/s, at 20Hz, I calculated the propagation is about 1.44deg going through a 1m long cable.

With 500,000,000m/s the signal takes 2ns/m
A fullwave of 20Hz takes 50ms
Thus the phase delay is: 360° x 2ns/m / 50ms = 14.4u° / m.

But the audible phase shift is related to other frequencies, because one can not hear an absolute delay.

Now if the reference signal travel with 1,100,000,000m/s this means they take: 0.9ns/m.
The difference to the 20Hz signal is 1.1ns/m.
The phase shift is 7.9u°/m.

*******
Not the best audio crossover filters come close to this values. They are decades away.
The phase shift in speakers (differece between different frequencies) is decades away.
Taking the travel speed of sound in the air into account this means we are speaking about.
(2ns/m, 300m/s) we are talking about 600nm (at 1 m cable).
This is decades away from any mechanical tolerances...

*****
To be honest, I did not spend much time:
* but I did not found an IMPRESSUM at the ICONOCLUST site
* and I did not find any "iconoclust" on the BElden site.

To me it seems that they maybe buy cables from Belden, maybe even good cables.
But they are not related to Belden somehow.
The whole document is just "marketing nonsens" in my eyes.

I wouldn't be surprised if BELDEN dissociates from this document.

Klaus

 

[BradtheRad]

Radio-Electronics magazine did an article about their analytical tests done to compare 'beastie' cables with plain wire.

The cable resistance is made
up of three components: the con-
tact resistance, the ohmic resis-
tance of the wire, and any
contribution from skin effect.

They found practically no difference in frequency response until they tested at 50 kHz, which is higher than the range of human hearing.

https://archive.org/stream/radio_electronics_1991-02/Radio_Electronics_February_1991_djvu.txt

The article starts about halfway down the page. Gibberish characters appear here and there. No illustrations.

 

[FvM]

The frequency dependency can be derived from these transmission line properties

see https://en.wikipedia.org/wiki/Transmission_line

The corner frequency is where R = jωL.

The question of practical interest is, when do these effects matter at all. For audio cables below several km length, the cable can be modelled as lumped circuit, series R and L, shunt C. G can be ignored in this context. The strongest effect is for low impedance speaker cables where R and L matters starting at a few meters. But you don't need complicated math to calculate it.

As KlausST has pointed out, the phase shift caused by the propagation speed variation in audio range is negligible for short cables, e.g. < 100 m.