snishanth512
Full Member level 3

Why are we using positive feedback for low-pass and high-pass filter?
Follow along with the video below to see how to install our site as a web app on your home screen.
Note: This feature may not be available in some browsers.


Do we? Can you please give an example of the circuit topologies you are talking about.Why are we using positive feedback for low-pass and high-pass filter?


HiWhy are we using positive feedback for low-pass and high-pass filter?
Do we? Can you please give an example of the circuit topologies you are talking about.

Why are we using positive feedback for low-pass and high-pass filter?

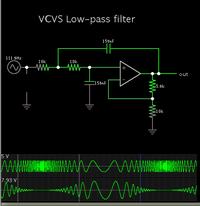


Why are we using positive feedback for low-pass and high-pass filter?

