newgirl
Newbie level 3

- Joined
- Feb 25, 2008
- Messages
- 3
- Helped
- 0
- Reputation
- 0
- Reaction score
- 0
- Trophy points
- 1,281
- Activity points
- 1,313
vrms formula
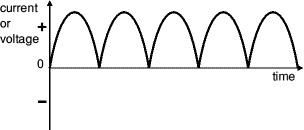
can someone give me a link on where i can get the relationship between VRMS and VDC( given rectified signal below) or are the the same ?
hxxp://www.kpsec.freeuk.com/images/dcvary.gif
thank you
can someone give me a link on where i can get the relationship between VRMS and VDC( given rectified signal below) or are the the same ?
hxxp://www.kpsec.freeuk.com/images/dcvary.gif
thank you