powersys
Advanced Member level 1

lm741 bandwidth
The circuit shown is a 2nd-order high-pass active filter using LM741. The frequency response of the filter is given in another figure below. Why does the gain (dB(Vo/Vin) of the filter start to drop again after 100kHz? For an ideal high-pass active filter, the gain after cut-off frequency should remain constant, right? Please advise. Thanks.
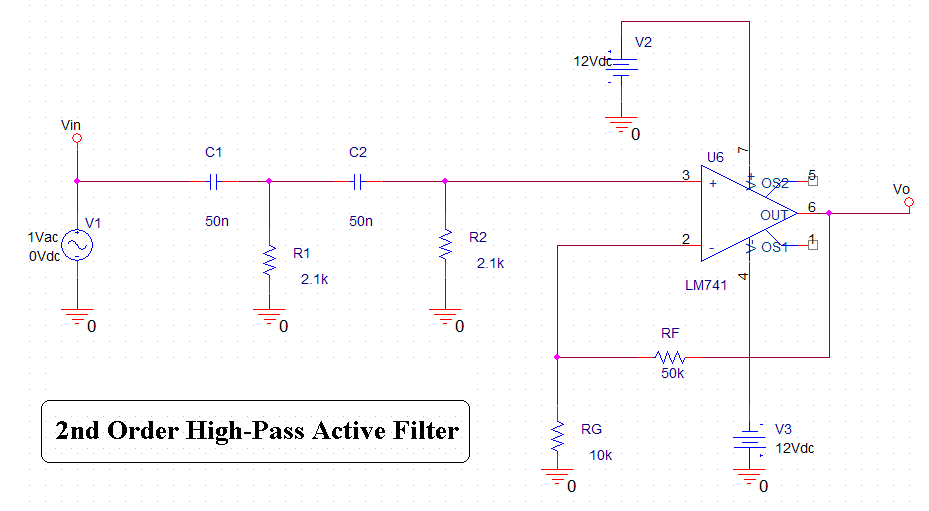
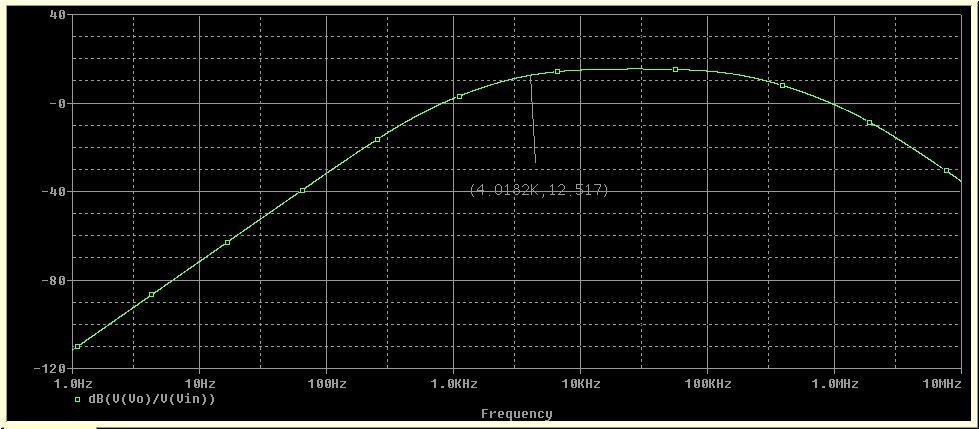
The circuit shown is a 2nd-order high-pass active filter using LM741. The frequency response of the filter is given in another figure below. Why does the gain (dB(Vo/Vin) of the filter start to drop again after 100kHz? For an ideal high-pass active filter, the gain after cut-off frequency should remain constant, right? Please advise. Thanks.




