zmkm1302022
Junior Member level 1

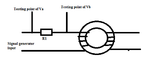
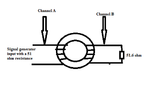
i cannot understand what is the characteristic impedance of a transformer used for, is it just for finding the leakage inductance and magnetizing inductance at different frequencies??