baby_1
Advanced Member level 1

hello
why and oscillator fix on specific value or how?
because the use positive feedback and the amplitude should increase and increase not fix how it fix?
example circuit
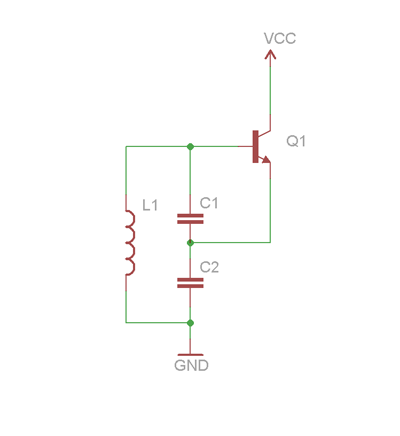
why and oscillator fix on specific value or how?
because the use positive feedback and the amplitude should increase and increase not fix how it fix?
example circuit







