This article is intended to be a quick guide on calculation of transformers used in AC voltage stabilizers which employes series topology. Such model is rarely used in low power equipments due to the relatively more complex control system required for drive the switching devices, but is specially suitable for employing at high power equipments, on what the weight of a conventional transformer (multi-taped) would be prohibitive, besides the fact that it reaches better resolution in the output compared to the standard approach.
A case study will be a stabilizer designed to handle an input ranging on ± 15% with a resolution of 1%.
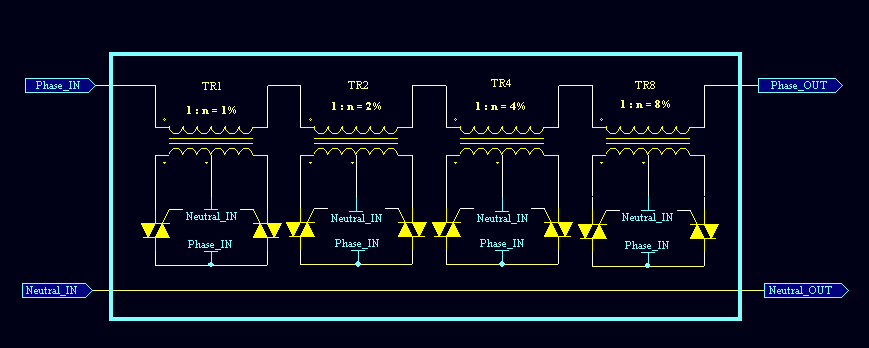
An illustration of the circuit is shown below, where we initially note the presence of 4 transformers:
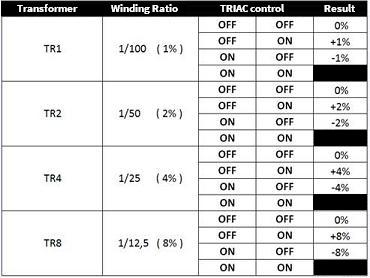
Basically, it performs a sum or subtraction of voltage by a binary logic, as follows:
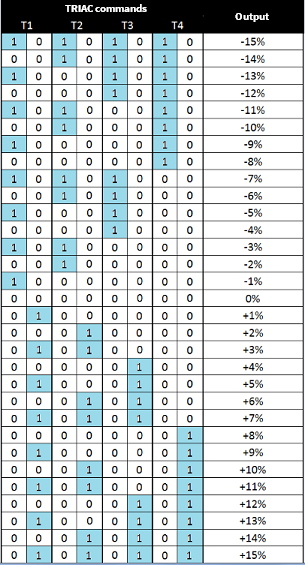
The table bellow show the logic required for the 8 switches working together:
An interesting aspect of this approach is that instead of working with a single transformer leading 100% of the power, we now have 4 smaller transformers, each one handling respectively 8%, 4%, 2% and 1% of the total power, which means that the total weight of the equipment will be substantially reduced. This weight reduction can be estimated by the following analysis:
The voltage on each transformer VTR is proportional to its turns ratio, and if we express the equation as a function of percentage, we obtain:

( 1 )
Knowing that the current flowing in the output is the same at transformers, we can apply the formula P = VI, as follows:

( 2 )
In order to obtain a relationship between dimensions and power of transformer ( in the case, of iron-silicon ) we can apply the well known equation which defines the cross-sectional area of the core as function of the power driven by its magnetic flux, as follows:
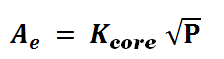
( 3 )
Since the focus here is only to present a comparison with the original topology, the constant value above does not matter, because will be eliminated on equation below, which provides the ratio of the cross sections of two topologies:
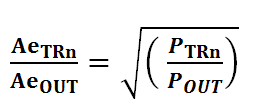
( 4 )
We can replace the variable PTR of the equation ( 4 ) by the value equation ( 2 ), obtaining the result:
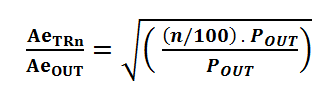
( 5 )
For the sake of clarity, in the following steps, we will refer to this relation by constant below:
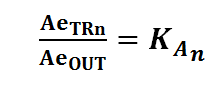
( 6 )
The result will be calculated for each transformer, yielding the following:
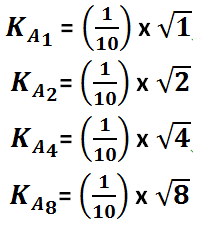
( 7 )
However, the section of the transformer itself does not bring us too much information about its weight.
We know that there is a relationship between the area and volume of 2 objects linearly proportionals:
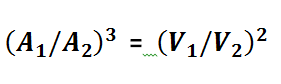
( 8 )
Just as before, for clarity purpose, we can identify the volume ratio by the following constant:
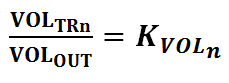
( 9 )
Applying constants ( 6 ) and ( 9 ) inside equation ( 8 ), yields:
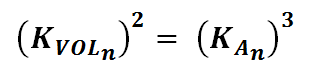
( 10 )
Calculating the values of equations ( 7 ) and ( 9 ) above results in the table below:
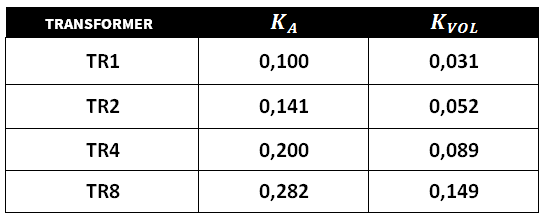
Note that the sum of the last column generates the following result:
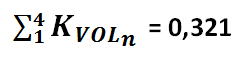
( 11 )
Conclusion :
For the above case study, the total weight for the four transformers employing serial topology is ~ 1/3 than would be required in standard topology, based on multi-tap.
A case study will be a stabilizer designed to handle an input ranging on ± 15% with a resolution of 1%.
An illustration of the circuit is shown below, where we initially note the presence of 4 transformers:
Basically, it performs a sum or subtraction of voltage by a binary logic, as follows:
The table bellow show the logic required for the 8 switches working together:
An interesting aspect of this approach is that instead of working with a single transformer leading 100% of the power, we now have 4 smaller transformers, each one handling respectively 8%, 4%, 2% and 1% of the total power, which means that the total weight of the equipment will be substantially reduced. This weight reduction can be estimated by the following analysis:
The voltage on each transformer VTR is proportional to its turns ratio, and if we express the equation as a function of percentage, we obtain:
( 1 )
Knowing that the current flowing in the output is the same at transformers, we can apply the formula P = VI, as follows:
( 2 )
In order to obtain a relationship between dimensions and power of transformer ( in the case, of iron-silicon ) we can apply the well known equation which defines the cross-sectional area of the core as function of the power driven by its magnetic flux, as follows:
( 3 )
Since the focus here is only to present a comparison with the original topology, the constant value above does not matter, because will be eliminated on equation below, which provides the ratio of the cross sections of two topologies:
( 4 )
We can replace the variable PTR of the equation ( 4 ) by the value equation ( 2 ), obtaining the result:
( 5 )
For the sake of clarity, in the following steps, we will refer to this relation by constant below:
( 6 )
The result will be calculated for each transformer, yielding the following:
( 7 )
However, the section of the transformer itself does not bring us too much information about its weight.
We know that there is a relationship between the area and volume of 2 objects linearly proportionals:
( 8 )
Just as before, for clarity purpose, we can identify the volume ratio by the following constant:
( 9 )
Applying constants ( 6 ) and ( 9 ) inside equation ( 8 ), yields:
( 10 )
Calculating the values of equations ( 7 ) and ( 9 ) above results in the table below:
Note that the sum of the last column generates the following result:
( 11 )
Conclusion :
For the above case study, the total weight for the four transformers employing serial topology is ~ 1/3 than would be required in standard topology, based on multi-tap.