htg
Full Member level 5

- Joined
- May 1, 2010
- Messages
- 248
- Helped
- 5
- Reputation
- 10
- Reaction score
- 3
- Trophy points
- 1,298
- Location
- Poland
- Activity points
- 2,862
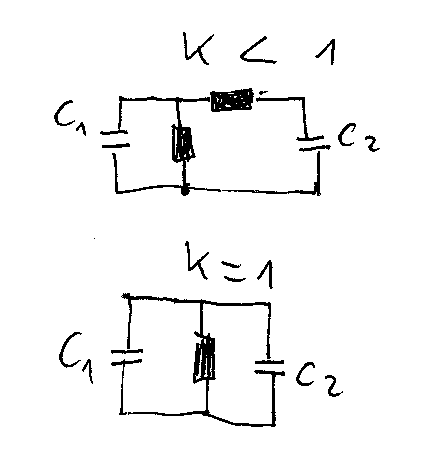
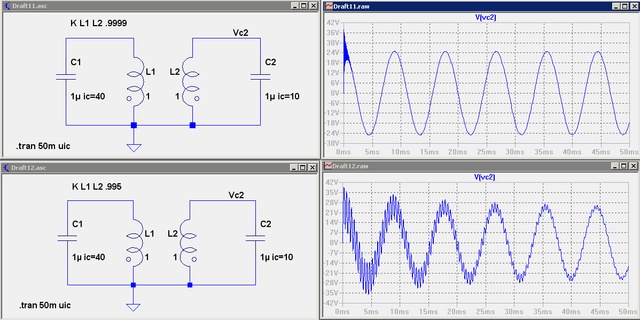
I consider two identical LC circuits, inductively coupled with the coupling coefficient k=1. Let y1 be the charge on the capacitor of the first circuit, y2 - charge on the capacitor of the second circuit. Let y1(0) = q > 0, y2(0) = 0. The equations that I get are y1'' + y2'' + ω^2 * y1 =0, y1'' + y2'' + ω^2 * y2 =0, where ω^2 = 1/(LC).
I subtract the second equation from the first and I obtain ω^2 * (y1-y2)=0, which contradicts the initial conditions y1(0)=q>0, y2(0)=0.
I do not understand why this problem appears and what to do.
I subtract the second equation from the first and I obtain ω^2 * (y1-y2)=0, which contradicts the initial conditions y1(0)=q>0, y2(0)=0.
I do not understand why this problem appears and what to do.